By Victor Haghani and James White ¹

Most of us associate the maxim “A penny saved is a penny earned” with Benjamin Franklin, but what he actually said is far more insightful: “A penny saved is two pence clear”. By the time he penned this, in “Hints for Those That Would be Rich” of the 1737 Poor Richard’s Almanac, Franklin was an experienced businessman who understood the nature of risk and uncertainty. Although he didn’t elaborate on this pithy bit of advice, we think that he was trying to convey an idea much more profound than the simple identity expressed in the misquote. We believe he was getting at the notion that one risk-free penny saved is worth two pennies of expected but uncertain business income.

Ben Franklin was a man well ahead of his time: it wasn’t until about 230 years later that Paul Samuelson and Robert Merton arrived at the same conclusion using the tools of mathematical finance.² Starting with the standard set of assumptions³ of a risk-averse investor and a single risky asset, they showed that if the investment in the risky asset is “perfectly” sized, the investor should be equally happy with a riskless investment delivering half the expected excess return of the investment in the risky asset.⁴ We could also say that under these circumstances, the investment’s “risk-adjusted return” is equal to half its expected excess return.

When Fees Saved are Worth More than Uncertain Extra Expected Return

One intriguing implication of this idea is that 1% more in investment management fees requires more than 1% in extra expected return, if getting the extra expected return increases the risk the investor is bearing. Just how much extra expected return an investor needs in order to offset the certain cost of higher fees depends on how much extra risk the manager has to take. For example, say an investor is considering moving from an index fund into an actively managed fund that charges 1% more in fees. Also assume that the index fund has a risk of 16%, but the active fund has a higher risk of 19% because it’s trying to generate higher returns by holding a more concentrated portfolio of stocks. In this case, the active fund needs to have a pre-fee expected return about 2% higher than the index fund to make the investor indifferent between the index fund and the active fund, a modern-day illustration of Franklin’s maxim.⁵

Conclusion

Philadelphia’s most famous resident tirelessly promoted the virtues of efficiency and rational thought which contributed so much to America’s economic success. It’s inspiring to realize that some of his ideas wound up being proven correct using mathematical techniques developed over 200 years later. Ben Franklin was a successful entrepreneur and his insight about the relative value of risky versus risk-free sources of return was developed in that light, but we see that it still has high practical value today for investors and business owners alike.

Appendix: A Slightly Deeper Dive

If we start with a risky portfolio which follows a geometric random walk,⁶ and then graph the relationship between Expected Excess Return, Risk-Adjusted Return, and the Price of Risk, it looks like this:

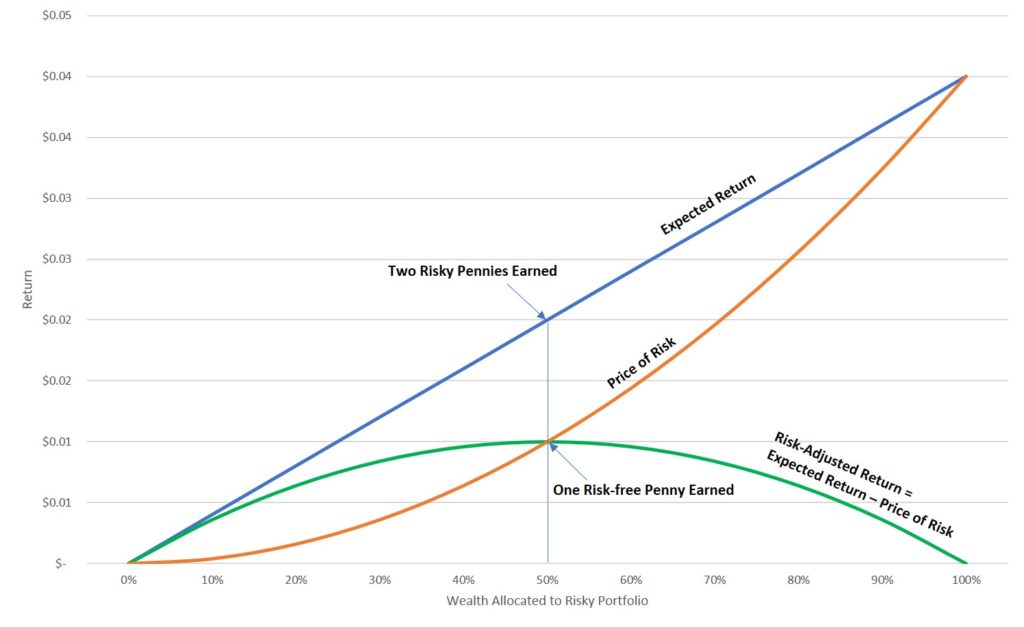
As the fraction of wealth invested in the risky portfolio increases, Expected Excess Return goes up in a straight line, while Risk-Adjusted Return goes up, peaks, then goes back down as risk starts to dominate. The optimal holding is the holding which maximizes Risk-Adjusted Return, or an allocation of 50% of wealth in this example.

Now we can also see that at the 50% point, Risk-Adjusted Return looks like it’s about half of the Expected Excess Return. In fact, it’s exactly half. In the theory of financial decision-making under uncertainty pioneered by Samuelson, Merton et al., there’s a result that within a standard set of assumptions the optimal Risk-Adjusted Return will always equal half of the Expected Excess Return at that optimal wealth allocation.⁷

So, to get one cent worth of Risk-Adjusted Return, the investor – regardless of their level of risk-aversion – would need two cents of Expected Excess Return if their wealth is optimally allocated. Risk-adjusted Return can be thought of as the risk-free return equivalent to the Expected Excess Return,⁸ so at the optimal allocation point we have a 2:1 ratio between the “risky” Expected Excess Return and the equivalent risk-free return.

1. Victor is the Founder and CIO of Elm Partners, and James is Elm’s CEO. Past returns are not indicative of future performance. This not is not an offer or solicitation to invest.
    

   Thank you to Larry Hilibrand, Vlad Ragulin and Jeff Rosenbluth for their helpful comments.
   

2. Samuelson and Merton weren’t the only ones or the first who arrived at this conclusion, but theirs is perhaps the most general formulation. See Robert C. Merton, “Lifetime Portfolio Selection under Uncertainty: the Continuous-Time Case,” The Review of Economics and Statistics (51), 1969, here.
   
3. The standard set of assumptions are that the portfolio consists of a single risky asset following Geometric Brownian Motion, the portfolio is continuously re-balanced, interest rates are constant, and the investor’s risk-aversion is consistent with iso-elastic utility (Constant Relative Risk-Aversion).
   
4. Where “perfectly” sized means sized to deliver optimal expected utility or optimal risk-adjusted return, and “equally happy” means to derive equal expected utility or equal risk-adjusted return.
   
5. In general, the relationship between fees and extra expected return needed to make the investor equally well-off will vary, as it is a function of how much extra risk the higher fee investment needs to take on to deliver the extra expected return. The lower the extra risk, the smaller the ratio will be (and if the higher fee investment has a lower risk, it can have a lower expected excess return).
    

   Also, the example assumes that the investor will move his holding to a new optimal asset allocation reflecting the new risk and return characteristics of the actively managed investment. The ratio between $1 of risk-free marginal return and the equivalent risky expected excess return may not be exactly 2:1 as in our stylized example, but it is generally valid that we should give extra weight to a source of risk-free return- the penny saved- compared to a risky source of extra expected return- the two pennies earned.
   

6. And the investor has a standard form of risk-aversion (CRRA), and re-balances the portfolio continuously.
   
7. For a more detailed mathematical treatment, see Appendix C of our note here.
   
8. Because an investment with a 1% risk-free return will also have a 1% Risk-Adjusted Return.